The Bondage Knots table
|
|
|
|
Fig. b : Irregular
|
|
Fig. c : Regular
|
|
|
As already discussed in a previous chapter the number of branches seems to be the incremental value that we shall use to classify the knots on a "Complexity axis".
You would certainly agree that a knot with 5 branches is more complex than another one having only 3. We shall then rank the knots by complexity along a vertical axis (Fig. a)
But what about comparing 2 knots that have the same number of branches?
This takes us back to the observations we've done before on the "shibari" knot : For a given number of branches one has first to differenciate irregular (Fig. b) and regular knots (Fig. c) .
Irregular knots will require specific description. They will not easily fit inside a table. We shall then keep them separate from the regular knots; although the complexity criteria will still apply to them.
|
Then, we have to find a way to differenciate regular knots of same complexity.
Hopefully this problem is quite simple. In the previous chapter we also saw how to build symmetrical (i.e. regular) knots. Numbers naturally describe rope's inputs and outputs; we shall use those numbers to sort the regular knots.
As an example for any complexity level, the first knot to be considered shall be the one with a rope entering on the left hand side of a branch (let's say branch 1) and exiting from the right hand side of the same branch. (Fig. d)
The knot immediately next to this one should be the one with a rope entering on the left side of branch 1 and exiting from the right side of branch 2. (Fig. e) And the next one with a rope entering on branch 1 and exiting from branch 3... etc...
|
|
|
Fig. d : Knot 1 for order 4 |
|
|
|
Fig. e : Knot 2 for order 4 |
|
Now our very task shall be to find the physical expression of the knots that we have just theoretically sorted; and we shall do that picking the appropriate specimens from the mathematical tables. Easy one. A
tutorial to help you tie some of the regular knots is available
here.
 |
|
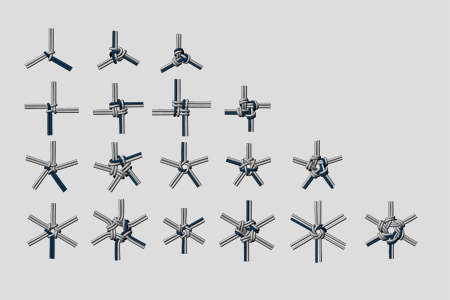
The principle of knots categories should be obvious to you now. A different number of branches on each row. The column index (starting at 0) is the shift between entering and exiting branch.
Those knots are called "regular" beacause the branches are symmetric by a rotation around the center of the knot.
In regular knots a rope "entering on the left" of a branch, "exits from the right" of the other branch. Otherwise, the symmetry would be broken and the knot would be called irregular.
|
Not all the knots that we need can be found in the Torus Knots family I conceed. It took me several years to find one physical knot of each kind. But now I'm very pleased to propose you this table. We will see together how we can use it to its best, combining all the results that we've come up with so far. Notice that these knots seem to be turning "to the right". The same knots exist which actually turn to the left. The actual number of physical knots that you have to know to complete your regular knots library is around 40.
Knots are closed
Mathematics classify the knots. The resulting tables have the drawback not to be immediately relevant to bondage riggers. But they also have the advantage to be comprehensive. Let us check very quickly which of the knots could be useful to us. The contribution of mathematics here is immediate. One can see at first sight that complex and odd knots are of no interest while one particular family seems to fulfill one important requirement: elegance and symmetry.
The table here to the side was produced with Knotplot (c). I really recommend you purchase this shareware if you want to dig further into this topic.
"Torus knots" are obviously of great interest.
Because the knots have to hold, very simple Torus knot of order 3 will not be considered. Neither the ones of order 7 and higher orders because they would be complex and not so elegant.
In the Torus Knots family, those of order 4 and 5 are definitely some knots that we will be using.
| |
 |
A major difference between mathematical knots and our day to day knots is the number of ropes. In mathematics, knots are closed and mostly made of one single rope. One could obviously transform such mathemaical knot into a physical one. As shown here below we just need to open them to make them feasible with one or more ropes.
|
Fig. c : Purely mathematical knot. Identified by numbers with no defined physical shape |
|
|
|
Fig. d : Closed loop theoretical view of the same knot. |
|
|
|
Fig. e : Physically doable form of the knot, made of multiple ropes. |
|
Macro links
Although Complexity (number of ropes) and Orientation (the way an entering rope exits the knot) remain the sorting criteria for our knots, it is worth to expose some hidden information that will be helpful in our bondages : The number of groups.
When drawn in their mathematical form (i.e. closed) the complex knots can be seen as macro links that one can chain to obtain a finished bondage. I would like you to play with the table and try to figure out which ones are made of one rope and which ones are made of several. Just imagine you did not connect the knot to anything but itself. Each branch would then be closed, thus defining sub-groups of closed loops within the knot.
In some cases, the knot itself would become one single macro link but in some other cases you will end up with 2,3,4, (...) groups. As an example observe this knot which is actually the sum of 2 separate closed loops. |
|

|
You need to understand that each of the groups can be treated as one link. The connection algebra will apply to them and you can rely on the complementary relationships to connect them without splitting them into more ropes.
Substitutions
Using the knots table is quite easy. Just pick the knot you need and you're good to go... But there is actually much more than this in this table than the ranking itself.
This is where things begin to be interesting. The challenge is not to fill the table with one knot of each kind but rather to rank all the knots you know and find as many as possible. Because the ultimate way to use this table is to define substitutes. Knots of the same kind can be replaced by one another. See an example of potential substitution here to the right. |

|

|
You shall then use the table to select the very best knot for your application. The entire purpose of this table is to serve the beauty of your ropework. Substitutions are a very nice way to control the design of your rope path. Of course the table can do much more : It will help you to visualize the rope path, define symmetries, insert loops, merge bondage parts that were designed separately...

















